Explanation of Coulomb's Law: Electrically Charged Objects. Also, Historical Quote.

The French engineer Charles Coulomb investigated the quantitative relation of forces between charged objects during the 1780's. Using a torsion balance device, created by Coulomb himself, he could determine how an electric force varies as a function of the magnitude of the charges and the distance between them.

Coulomb used little spheres with different charges whose exact value he did not know, but the experiment allowed him to test the relation between the charges. Coulomb realized that if a charged sphere touches another identical not charged sphere, the charge will be shared in equal parts symmetrically. Thus, he had the way to generate charges equal to ½, ¼, etc., from the original charge. Keeping the distance constant between the charges he noticed that if the charge of one of the spheres was duplicated, the force was also duplicated; and if the charge in both spheres was duplicated, the force was increased to four times its original value. When he varied the distance between the charges, he found the force decreased in relation to the square of the distance; that is, if the distance was duplicated, the force decreased to the fourth part of the original value.

In that way Coulomb demonstrated that the electric force between two stationary charged particles is :
- Inversely proportional to the square of the distance r between the particles and is directed along the line that joins them.
- Proportional to the product of the charges q₁ and q₂.
- Attracted if the charges have opposite electrical sign and repulsed if the charges have equal sign.

Coulomb 's Law can be expressed in the form of an equation :

The validity of Coulomb's Law has been verified with modern devices that have detected that the exponent 2 has an exactitude of one part in 10¹⁶.

K_e is a constant known as the Coulomb 's constant, which in the International System units has the value K_e = 8.987x10⁹ Nm²/C².

The International System unit for charge is the Coulomb.
The smallest known charge in nature is the charge of an electron or proton, which has an absolute value of e = 1.60219x10^-19 C.
Thus, a 1 Coulomb charge is approximately equal to a charge of 6.24x10¹⁸ (= 1C/e) electrons or protons.

We should notice that the force is a vectorial quantity, that is, has magnitude and direction. Coulomb 's Law expressed in vectorial form for the electric force F₁₂ exerted by a charge q₁ over a second charge q₂ is (bold type is used to denote vectorial quantities):

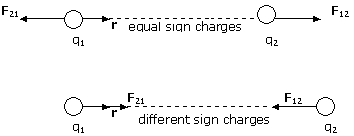
As every force obeys Newton's third Law, the electric force exerted by q₂ over q₁ is equal in magnitude to the force exerted by q₁ over q₂ and in the opposite direction, that is F₂₁= - F₁₂.

If q₁ and q₂ have the same sign, F₁₂ takes the direction of r. If q₁ and q₂ have opposite sign, the product q₁q₂ is negative and F₁₂points opposite to r.

When two or more charges are present, the force between any pair of them is given by the above equation. Hence, the resultant force on any of them is equal to the vectorial sum of the forces exerted by the different individual charges. For example, with three charges, the resultant force exerted by particles 2 and 3 over 1 is

F₁ = F₂₁ + F₃₁